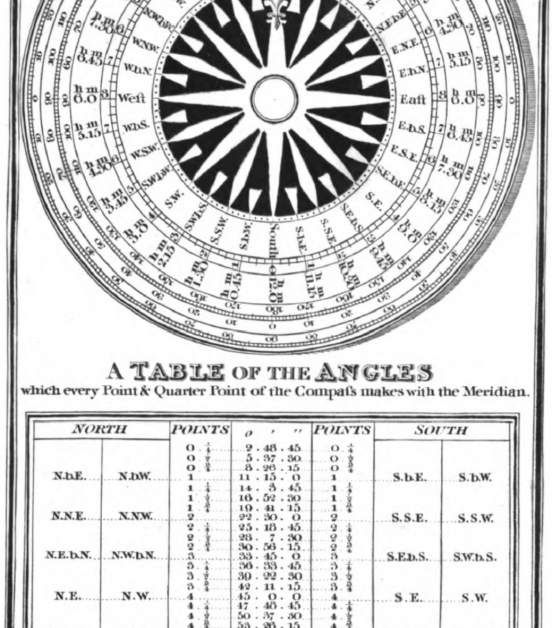
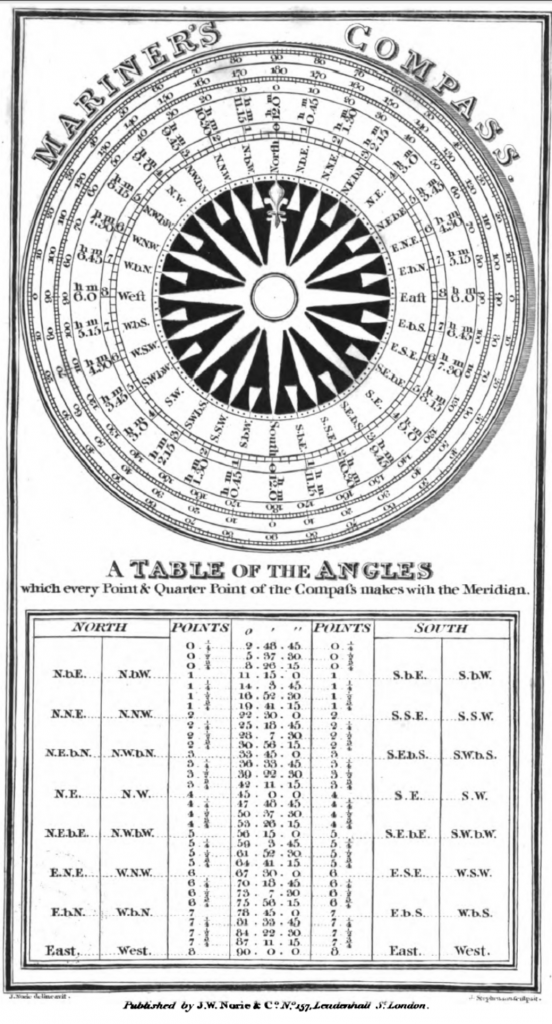
The magnetic compass has developed, not as quickly as one might think, over the last seven or eight centuries. Mariners have been navigating the seas out of sight of land for much longer than this: directions had to be based on the bearings of the Sun and stars although local use could be made of prevailing wind directions such as the Italian Tramontana for North. The use of known wind directions in different cultures evolved versions of a card with four then eight named directions. The use of local winds for directions, such as Boreas or Tramontana for North, was common in the Mediterranean but it was the North Sea cultures who adopted Cardinal points. It may have been King Alfred who introduced the inter cardinals such as NW. 1King Alfred – See Gurney, Alan. Compass: A Story of Exploration and Innovation. 1st ed. New York: Norton, 2004.
Today we mix two systems of direction. The older system is where the circle is repeatedly bisected between the Cardinal points of the compass. The modern is the, in some ways more convenient, division of the circle into 360 parts: this goes back to ancient times and forms the basis for our division of time of day.
The use of repeated halving is common in the development of older measuring systems: division by twos gives four, eight, sixteen and thirty-two parts. These are called “points” making one point equivalent to 360/32 or 90/8 = 11¼º. 2This, of course, explains the visibility angle of navigation lights being two points abaft the beam at 112½º which otherwise looks a bit odd. Later iterations of the card went as far as 128 divisions using quarter and half points. These divisions are created in the following manner. For clarity only one quadrant is illustrated as the principles are the same:
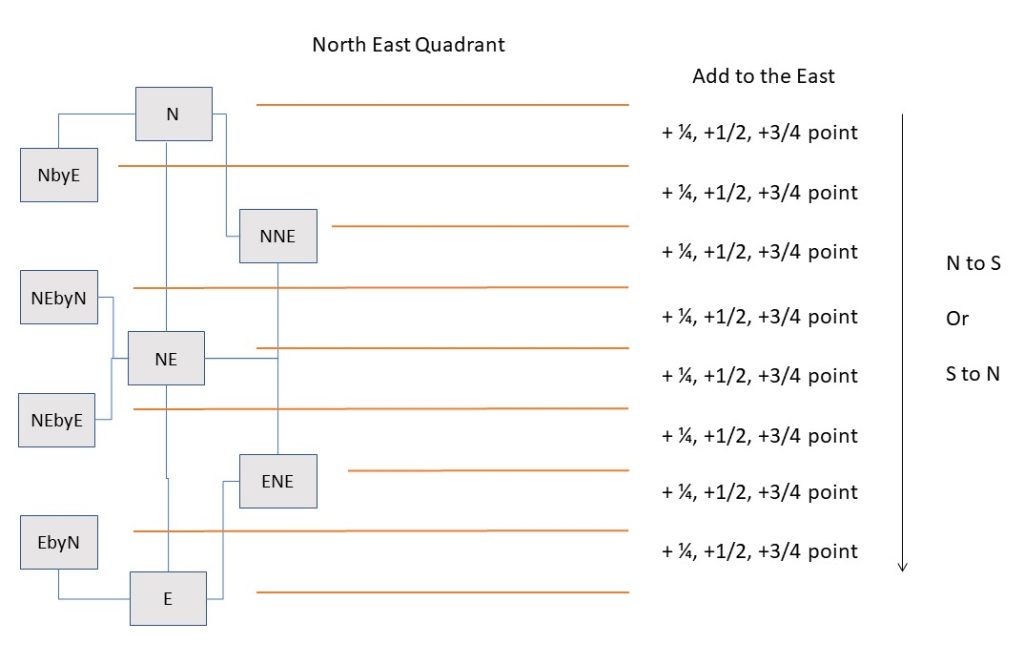
Cardinals and inter Cardinals – the convention is to use eight directions. These are Cardinals N,S,E,W with the inter Cardinals formed by adding adjacent Cardinals. This gives another four directions NE, SE, SW, NE .
Halving – There are then eight sub directions made in the gaps between the Cardinals and inter Cardinals. These are referenced by the name of the nearest Cardinal/inter Cardinal with “by” and the name of the other Cardinal in the quadrant. This gives a description such as N by NE (NNE) or E by NE (ENE). Adjacent to a Cardinal it would be, say, E by N (being the other Cardinal). This makes a total of sixteen directions spaced apart by 360º/16= 22½º.
Quartering – as there is still an angle of 22½º between any two divisions we subdivide again to get sixteen more directional “points” in the gaps. These take each of the eight Cardinals/inter Cardinals such as N or NW and create a direction on each side. We add the name of the next nearest cardinal in that quadrant such as N by W or NW by W. We now have thirty two directions or “points” of 11¼º. Note that all of these names are combinations of Cardinals and inter Cardinals and that, provided the rule is understood, any named point can be easily placed.
Each 11¼º point – is considered as covering the space 5 1/8 º either side and so is not very precise for modern use. This used not to matter as there were so many sources of uncertainty given that bearings, variance, deviation and other factors were imprecise. These problems were resolved by British sailors and scientists such as Halley, Cook, Flinders, Thompson and Airy leading to better instruments and charts. It was then desirable to move to 64 and then 128 directions.
1/4 points – extra directions were made not by inventing further points but by appending one, two, or three quarter points (11½º/4= 2.8125º) to each of the thirty two directional points above. These were named by the adjacent point and moving southwards from North or northwards from South to East or West in steps of 1/4 point (2.8125º). Put another way we always move from the poles of the compass (N or S) towards the equator (E or W). As there are 32 points these further divisions make a total of 32×4 = 128 directions with a resolution of 2.8125º. Using this system, the space between each the thirty two points is filled. All the divisions in each quadrant will only use the two Cardinals of that quadrant. This is still imprecise as each of the 128 divisions covers 3°.
As an example of one of these fine divisions we could take, say, NW by W 3/4 W (NW=315, NW by W is one point West so subtract 11.25 = 303.75). then subtract 3/4 of 11¼º giving 295°.3125. The next point would be W by NW (292.5).
Amplitude – Even when converted to degrees, not easy with the fractions, this gives a quadrantal direction or “Amplitude” such as S 55º W not 235º as we would use today. This usage is found in the Sun rise/set amplitude tables in the Almanac but generally not seen in normal use. Obviously the 128 points were unwieldy so eventually there was a switch to the more convenient 0-360º system we use today.
In the age of sail, it was necessary to learn the thirty-two points by rote 3See 1853 The Young Sea Officer’s Sheet Anchor by Darcy Lever and be able to know how to move between them. For example, a ship could generally sail no closer than six points to the wind so it would be necessary to know the point of sail on the opposite tack or what was one or two points free of the wind. Learning this was known as “Boxing the compass”. 4Boxing the Compass – a good explanation here. In time sailors would acquire a sense of a point or fraction of one – “sail two points on the larboard quarter”. Whilst not requiring a great deal of numeracy the “Points” system did need greater memory and visualisation skills than might be found in today’s sailors.
However, the terms still need to be understood when reading old sailing directions and trying to relate them to modern charts (why not buy an up to date pilot? – you may ask). Either system works on its own terms but they are difficult to combine. Having gained an understanding of the system the easy solution is to use a conversion table. 5Conversion table – Extract from Bowditch It would have been easier if we had adopted either the Chinese practice of division by 24 or even 36. giving a 15º or 10º point. But we didn’t.
A legacy of the Italian compass rose was the use of “T” (Tramontana) for North and a cross for East representing Jerusalem. 617C examples in The Sea Chart by John Blake The “T” has survived as a Fleur de Lys. Note that the Italians retained their wind names for directions long past everybody else, they also have an idiosyncratic approach to port and starboard. The “Rose” associated with the compass comes from Portuguese usage.
Notes and References
Conversions
The closest calculator found is this one.
- 1King Alfred – See Gurney, Alan. Compass: A Story of Exploration and Innovation. 1st ed. New York: Norton, 2004.
- 2This, of course, explains the visibility angle of navigation lights being two points abaft the beam at 112½º which otherwise looks a bit odd.
- 3
- 4Boxing the Compass – a good explanation here.
- 5Conversion table – Extract from Bowditch
- 617C examples in The Sea Chart by John Blake
- 7Norie, John William. A Complete Set of Nautical Tables. author, and sold, 1836.
Image Credits and Sources
- 1836 Nories tables compass card: 1836 Nories Tables